https://doi.org/10.1140/epjp/s13360-020-00972-x
Regular Article
Entanglement induced by noncommutativity: anisotropic harmonic oscillator in noncommutative space
1
Department of Physical Sciences, Indian Institute of Science Education and Research Kolkata, 741246, Mohanpur, India
2
Physics and Applied Mathematics Unit, Indian Statistical Institute, 203 B.T. Road, 700108, Kolkata, India
Received:
20
July
2020
Accepted:
28
November
2020
Published online:
4
January
2021
Quantum entanglement, induced by spatial noncommutativity, is investigated for an anisotropic harmonic oscillator. Exact solutions for the system are obtained after the model is re-expressed in terms of canonical variables, by performing a particular Bopp’s shift to the noncommutating degrees of freedom. Employing Simon’s separability criterion, we find that the states of the system are entangled provided a unique function of the (mass and frequency) parameters obeys an inequality. Entanglement of Formation for this system is also computed and its relation to the degree of anisotropy is discussed. It is worth mentioning that, even in a noncommutative space, entanglement is generated only if the harmonic oscillator is anisotropic. Interestingly, the Entanglement of Formation saturates for higher values of the deformation parameter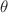 
, that quantifies spatial noncommutativity.
© Società Italiana di Fisica and Springer-Verlag GmbH Germany, part of Springer Nature 2021
