https://doi.org/10.1140/epjp/s13360-020-00960-1
Regular Article
On edge irregular reflexive labeling for Cartesian product of two graphs
1
Naval University of Engineering, 430033, Wuhan, China
2
Centre for Advanced Studies in Pure and Applied Mathematics, Bahauddin Zakariya University, Multan, Pakistan
3
Department of Mathematics, COMSATS University Islamabad, Lahore Campus, Lahore, Pakistan
Received:
6
November
2020
Accepted:
20
November
2020
Published online:
1
January
2021
A graph labeling is an algorithm that assignment the labels, traditionally represented by integers, to the edges or vertices, or both, of a network(graph) G. Formally, given a graph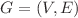 
, a vertex labeling is a function of V to a set of labels. A graph with such a function defined is called a vertex-labeled graph. Likewise, an edge labeling is a function of E to a set of labels. In this case, the graph is called an edge-labeled graph. We study an edge irregular reflexive k-labeling for the Cartesian product of two paths, two cycles and determine the exact value of the reflexive edge strength for the Cartesian product of two paths and two cycles.
© Società Italiana di Fisica and Springer-Verlag GmbH Germany, part of Springer Nature 2021
