Lie group analysis, analytic solutions and conservation laws of the (3 + 1)-dimensional Zakharov-Kuznetsov-Burgers equation in a collisionless magnetized electron-positron-ion plasma

Xia-Xia Du; Bo Tian; Xiao-Yu Wu; Hui-Min Yin; Chen-Rong Zhang

doi:10.1140/epjp/i2018-12239-y

EPJ

Lie group analysis, analytic solutions and conservation laws of the (3 + 1)-dimensional Zakharov-Kuznetsov-Burgers equation in a collisionless magnetized electron-positron-ion plasma

Xia-Xia Du, Bo Tian^*, Xiao-Yu Wu, Hui-Min Yin and Chen-Rong Zhang

State Key Laboratory of Information Photonics and Optical Communications, and School of Science, Beijing University of Posts and Telecommunications, 100876, Beijing, China

Received: 23 February 2018
Accepted: 9 August 2018
Published online: 21 September 2018

Abstract

We work on the (3+1)-dimensional Zakharov-Kuznetsov-Burgers equation for the ion-acoustic waves in a collisionless magnetized electron-positron-ion plasma. We derive the Lie point symmetry generators and Lie symmetry groups. We construct certain solutions which are related to the known solutions. Using the symmetry generators, we obtain the reduction equations, through one of which we derive some power-series solutions and travelling-wave solutions including the shock solutions via the power-series and polynomial expansion methods. Shock waves are pictured out. 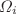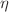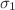Effects of the normalized ion gyrofrequency, $\Omega_{i}$ , the normalized kinematic viscosity, $\eta$ , the real parameter measuring the deviation from the Maxwellian equilibrium, $\kappa$ , the ratio of the ion temperature to electron temperature, $\sigma_{1}$ , and the ratio of the electron temperature to positron temperature, 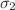$\sigma_{2}$ , on the amplitude of the shock wave, |a|, are found: i) |a| is a hyperbolic function of 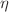$\eta$ ; ii) $\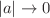vert a\vert \rightarrow 0$ when $\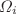Omega_{i}$ , $\kappa$ or $\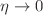eta \rightarrow 0$ ; iii) | a| goes to a constant when 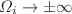$\Omega_i \rightarrow \pm \infty$ ; iv) |a| becomes a constant when 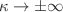$\kappa \rightarrow \pm \infty$ ; v) $\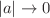vert a\vert \rightarrow 0$ when $\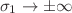sigma_{1} \rightarrow \pm \infty$ ; vi) |a| keeps unchanged when 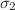$\sigma_{2}$ varies and $\sigma_{1}= \frac{-3(2\kappa + \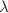lambda^{2} - 2\kappa\lambda^{2})}{5(-1+2\kappa)}$ where $\lambda$ denotes the ion-acoustic wave propagation speed; vii) 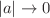$\vert a\vert \rightarrow 0$ when $\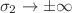sigma_{2} \rightarrow \pm \infty$ and $\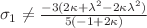sigma_{1} \neq\frac{-3(2\kappa +\lambda^{2}-2\kappa\lambda^{2})}{5(-1+2\kappa)}$ . We present the conditions of the nonlinear self-adjointness. 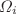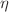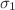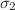Based on the nonlinear self-adjointness, we construct the conservation laws which are related to $\Omega_{i}$ , $\eta$ , $\kappa$ , $\sigma_{1}$ and $\sigma_{2}$ .

Student Conference in Optics and Photonics 2024
October 2-4, 2024
Barcelona, Spain

EPJ

Lie group analysis, analytic solutions and conservation laws of the (3 + 1)-dimensional Zakharov-Kuznetsov-Burgers equation in a collisionless magnetized electron-positron-ion plasma

Conference announcements