https://doi.org/10.1140/epjp/i2017-11453-5
Regular Article
Multi-soliton interaction of a generalized Schrödinger-Boussinesq system in a magnetized plasma
State Key Laboratory of Information Photonics and Optical Communications, and School of Science, Beijing University of Posts and Telecommunications, 100876, Beijing, China
* e-mail: tian_bupt@163.com
Received:
6
October
2016
Accepted:
13
March
2017
Published online:
27
April
2017
Under investigation in this paper is a generalized Schrödinger-Boussinesq system, which describes the stationary propagation of coupled upper-hybrid waves and magnetoacoustic waves in a magnetized plasma. Bilinear forms, one-, two- and three-soliton solutions are derived by virtue of the Hirota method and symbolic computation. Propagation and interaction for the solitons are illustrated graphically: Coefficients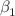 
and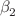 
can affect the velocities and propagation directions of the solitary waves. Amplitude, velocity and shape of the one solitary wave keep invariant during the propagation, implying that the transport of the energy is stable in the upper-hybrid and magnetoacoustic waves, and amplitude of the upper-hybrid wave is bigger than that of the magnetoacoustic wave. For the upper-hybrid and magnetoacoustic waves, head-on, overtaking and bound-state interaction between the two solitary waves are asymptotically depicted, respectively, indicating that the interaction between the two solitary waves is elastic. Elastic interaction between the bound-state soliton and a single one soliton is also displayed, and interaction among the three solitary waves is all elastic.
© Società Italiana di Fisica and Springer-Verlag Berlin Heidelberg, 2017
